A climb-glide routine can potentially yield a useful increase in range compared with the same airframe/propeller/motor combination operating straight-and-level at best range speed point or best endurance point respectively.
Let me explain briefly with graphs of the results how I have come to that conclusion, at least theoretically so far, hopefully including some useful detail on the method of my calculations and models. I intend to experimentally validate this concept at some point with my APM-powered Skyfun, so I have tailored this example with measured and guestimate data as best I can for this style and size of UAV. The propeller is oversized compared with what is normally run on the Skyfun (8" vs 5-6") due to the non availability of data at the smaller sizes.
Best Endurance Steady-state Flight
Firstly, we look at the airframe drag power curves as a function of airspeed. For this model a simple Prandtl model of an aircraft is used. The form drag power and induced drag power combine to form a "U"-shaped total drag power curve with a minima representing the best endurance point (lowest power consumption). The point where a tangent line to this drag power curve passing through the origin touches the curve, (or, in the case of head or tailwind components, with the origin point offset in the speed axis by the appropriate offset) yields the minimum energy consumption per unit distance - i.e. best range speed. This is all valid for steady-state, straight-and-level operation and is only considering aerodynamic power absorbed.
Adding in the propeller and electric motor models to the calculation, we can estimate the shaft power and electrical power consumed to overcome this aerodynamic drag. The propeller model is a regressed 4th order polynomial surface model based on UIUC propeller data The efficiency curve for an 8x6" APC-E propeller shown here shows how the polynomial model compares with the UUIC data. This model is actually two models - one for thrust coefficient and one for power coefficient. It isn't a perfect model by any stretch, but it allows an analytical solution for the overall modelling process.
The electric motor is modelled as a first-order model as described by Mark Drela
The motor Kv was estimated to give a voltage requirement for a 25% climb gradient at 28.5m/s of approximately 14.8V (4S battery pack assumption). This is somewhat arbitrary and the assumption does influence the motor efficiency somewhat, but it also gives a realistically useful top speed estimate of 33-34m/s. It is possible to optimise the motor efficiency in cruise a fraction further by manipulating the Kv value, but only by choosing to compromise climb and max speed performance. The motor model used yields a peak efficiency of a realistic 81%
Having modeled the propeller and the motor, these two power curves sit respectively above the aero drag curve by an amount representing the successive inefficiency of each of these processes. The minima of the electric power curve now yields the best electrical endurance speed and, likewise, the tangent point for best electrical range speed. Note that both points are at somewhat increased airspeeds - something to bear in mind even for straight and level operations!
Just for interest, here below are the propeller and motor efficiency curves for steady-state flight. It shows clearly some of the compromises of steady-state operation where the efficiency points of the electric motor and propeller are obviously not co-located. Notice too that increasing speed increases the total propulsion efficiency, excepting that the gain here is subsequently lost in airframe drag.
System Efficiency during Climb
If we now add a climb (vertical) velocity, then knowing the airframe mass (in this example specified at 1kg), we can quite easily calculate the additional power required to climb and add this to the net thrust power. Not forgetting to correct for the increased hypotenuse speed of the climb gradient, this generates a new thrust value the propeller needs to generate, hence a higher propeller speed, more shaft power and therefore more electrical power. This is shown in the following graph, where the best range climb point (curve minima) is clearly visible. A key point to understand is that much of the extra power expended is being stored in a potential energy "battery". (PEB). There are some subtleties here that I have not modeled, since increasing climb angle transfers some of the airframe lift duties to the propeller thrust. I initially attempted to do the vector math, but quickly threw it in the too-hard-for-now basket, however I may revisit this. I do suspect the effects are small and potentially favourable.
Note how the best range speed during climb is faster than at straight-and-level. This is useful because it compensates for the climb angle reducing the horizontal speed and also helps offset the slower best L/D speed during the glide period. In this example, as we will see in the calculations below, the net horizontal speed (but not necessarily ground speed) is within a bull's roar of the best range speed when straight and level.
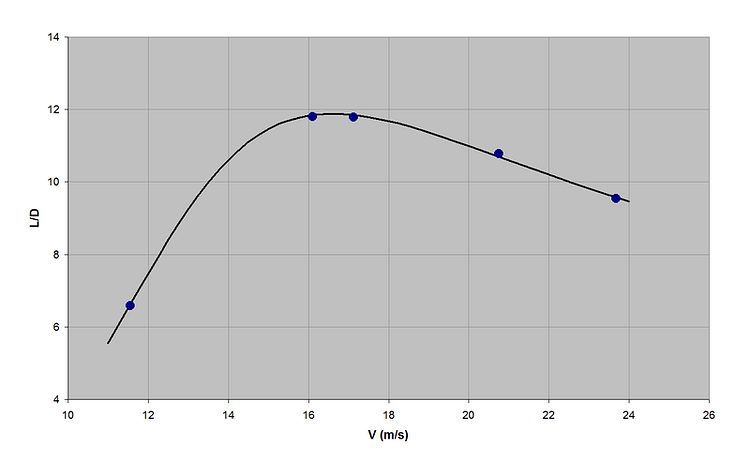
Glide Performance
For this modelling exercise, I have assumed, reasonably enough, that the glide mode will be at best glide ratio, which in this case is about 8.5:1. The second graph above shows the peak L/D to be coincident with the best range speed from the airframe drag power curve - no voodoo in that, really.
It's worth noting that optimal glide performance will only be achieved with a stopped propeller (or, ideally a folded propeller!). With a fixed propeller this will probably require the continuous use of the ESC brake function which may consume some power. Such are the differences between theory and practice.
Combining Climb and Glide Modes
For the horizontal speed calculations, we need to correct the airspeed to consider only the horizontal component to make valid comparisons with straight-and-level flight. Using the example of this study, this takes the best range climb speed of 18m/s at 15% gradient down to 17.46m/s horizontal speed and for the 8.5:1 glide, 15m/s to 14.89m/s. To compare directly with straight-and-level operation, if we take a climb-glide cycle, the average speed is 15.7m/s - fractionally slower than the 16m/s straight-and-level cruise. The electrical energy consumption is assumed to only occur during the climb phase, so factoring this over the whole climb-glide cycle (working in energy units/unit distance or J/m) gives an average energy consumption of 2.18J/km compared with 2.50J/km when travelling straight-and-level - a 12.7% decrease in energy consumption or a 14.6% increase in range.
This isn't actually the optimal case as calculated by this model. The 25% climb gradient yields a slightly better 15.2% increase in range, but since this model doesn't include the battery discharge efficiency, we can't be sure this really is optimal.
Note that it is now possible to trade this range gain off in the glide phase to recover or even increase the average speed over the straight-and-level condition. The choice is yours!
Where do the Efficiency Gains come from?
If we plot efficiency curves for propeller and motor together it becomes immediately clear that the vast majority of the efficiency gain is found in operating the electric motor closer to its peak efficiency. It must be emphasised that this is for this case only! Other airframe, motor and propeller combinations will move this around.
Indeed it opens the possibility of re-sizing the motor in lieu of climb-glide operation, however this will most likely limit the maximum power output of the motor, limiting climb rate and making take-off difficult without applying additional measures.
Summary
This was prepared for my own curiosity, but I hope that it can shed some light on the possibilities that relatively simple modelling can offer in helping to optimize a fixed-wing UAV. All this was done using MS Excel, including the regression analysis of the propeller model.
I am in no way claiming that climb-glide is the best solution for everyone's application. Merely that it is a way to improve the energy efficiency of the relatively simple hardware set that most people adopt for their UAV's.